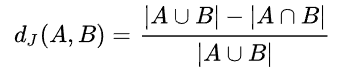Distance Calculation

---

---

Distance measures quantify the degree of separation or distance between two data points in a specific metric space.
The focus here is on determining how far apart two points are in terms of their coordinates, features, or representations.
Distance measures are always non-negative and are usually symmetric (i.e., the distance from point A to point B is the same as the distance from point B to point A).

 

What methods can we use?

 

---

Euclidean distance

 

2D space:

 

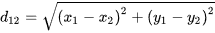
Full space:

 

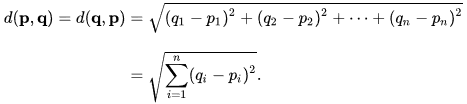
Standardized Euclidean distance

---

---

Manhattan distance

 

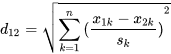
2D space:

 

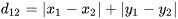
Full space:

---

---

Chebyshev distance

 

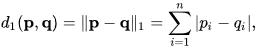
2D space:

 

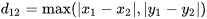
Full space:

---

---

Minkowski distance

 

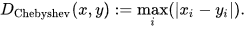
2D space:

 

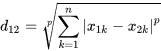
Full space:

---

---

---

---

---

---

---

---

---

 Hamming distance

 

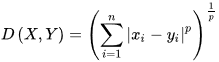
In information theory, the Hamming distance between two strings of equal length is the number of positions at which the corresponding symbols are different. In other words, it measures the minimum number of substitutions required to change one string into the other, or the minimum number of errors that could have transformed one string into the other.

 

---

---

Jaccard distance

 

 

---